Search the Community

Can Any provide guidance on designing a 14-story building in Etabs using flat slabs with a thickness of 30 cm? What checks are necessary for flat plate slab buildings? Also, how can I check the punching shear of slabs at column locations? If you have a reference file, please share it with me.

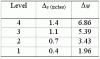
*SEFP Consistent Design* *UBC Seismic Drift Limits* *Doc No: 10-00-CD-0003* *Date: June 04, 2013* The goal of this tutorial is to demonstrate how to evaluate building drifts and story drifts using UBC 97 guidelines. The philosophy behind Story Drift Limits is “Deflection Control”; In UBC 97, deflection control is specified in terms of the story drift as a limit on the lateral displacement of one level relative to the level below. The story drift is determined from the maximum inelastic response, ΔM. Let’s start by defining the design-level response displacements. The elastic deflections due to strength-level design seismic forces are called design-level response displacements. These are denoted by ΔS, where the subscript ‘s’ stands for strength design. Design level response displacements are what you get out of your software, when you run analysis. Please note that structural analysis softwares may provide these values in different formats; say a percentage of height or a direct output. Well, to calculate your story drifts, first you need to find maximum inelastic response displacements from your design-level response displacements. The maximum inelastic response displacement is defined as: ΔM = 0.7RΔS Where, R is the structural system coefficient, the subscript ‘m’ in ΔM signifies that we are calculating a maximum value for the deflection due to seismic response that includes inelastic behavior. Seismic drift values are much larger than wind values. UBC uses maximum inelastic response displacements rather than the design level displacements to verify the performance of the building. Seismic drift limits are 2% & 2.5% of the story height for long and short -period buildings. For a floor to floor height of 12 feet the max., allowable inelastic drift value would be 2% of 12 feet= 0.02*12*12 in=2.88 in. For wind for a 12 story height, drift would be L/400=12*12/400 =0.36 inches, A comparison of both wind and seismic drift limits shows that earthquake inelastic displacements are quiet large compared to wind displacements. That is why proper detailing is emphasized in seismic design. When calculating ΔS for seismic, make sure: You have included accidental torsion in your analysis. Use strength design load combinations: 1.2D + 1.0E + 0.5L & 0.9D + 1.0E. You are using cracked section properties for reinforced concrete buildings. Typical values are Icr walls= 0.5EcIg, Beams = 0.5EcI g & for Columns 0.5 - 0.7 EcIg. Use a reliability/ redundancy factor= 1 to calculate seismic forces. Whenever the dynamic analysis procedure of §1631 is used, story drift should be determined as the modal combination of the story drift for each mode. Determination of story drift from the difference of the combined mode displacements may produce erroneous results because maximum displacement at a given level may not occur simultaneously with those of the level above or below. Differences in the combined mode displacements can be less than the combined mode story drift. Example: A four-story special moment-resisting frame (SMRF) building has the following design level response displacements.(See attached Image) R= 7.0, I= 1 Time period= 0.6 sec (See the attached image for Story Information) Calculate: Maximum Inelastic response displacements. Story drift in story 3 due to ΔM. Check story 3 for story drift limit. Maximum Inelastic response displacements ΔM = 0.7RΔS ΔM = (0.7) (7) ΔS = (4.9) ΔS (See the attached image for Maximum Inelastic response displacements) Story drift in story 3 due to ΔM Story 3 is located between Levels 2 and 3. Thus ΔM drift = 5.39 - 3.43 = 1.96 in. Check story 3 for story drift limit. For structures with a fundamental period less than 0.7 seconds, §1630.10.2 requires that the ΔM story drift not exceed 0.025 times the story height. For story 3: Story drift using ΔM = 1.96 in. Story drift limit = 0.025 *(12*12) in = 3.6 in. > 1.96 in. Therefore, Okay.

Asalamualaikum, Are seismic zones same for eurocode and UBC? In UBC, we have zone 4 as the high seismic zone. In Eurocode, do we have same seismic zones? I am doing risk assesment using ELER software, it have three seismic zones for European building, Zone 1,2 and 3... What is the PGA for Zone 1 2 and 3 for European building codes? I am attaching a picture found from the following website http://www.protezionecivile.gov.it/jcms/en/classificazione.wp stating that Zone 1 is most dangerous zone Thank you for the help

Under what conditions we should use load combinations mentioned under Subject heading in UBC -97? As per my research we should use them only for " Element supporting Discontinuous System"-UBC 97-1630.8.2 and for "Collector Elements"-UBC 97 1633.2.6. So, there is no need to use these combinations for all structures and its components (Exceptions are mentioned above). Is my understanding correct? Please reply with referrence